Mini
[알고리즘] 백준 11660 구간합 구하기5 // 2차원 누적합 본문
https://www.acmicpc.net/problem/11660
* 풀이
- data 정의를 잘해야함

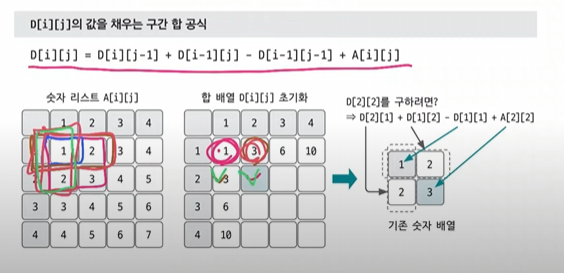
- 2차원 누적합 채우는 방법
- 위 + 왼 - 좌측위(중복덧셈) + 원본
- 3 + 3 - 1 + 3
- 쿼리 구하기
- x2,y2사각형 의 누적합에서 # sum(x2,y2)
- 범위밖 위 사각형을빼고 # sum(x1-1,y2)
- 범위밖 좌측 사각형을 빼고 # sum(x2,y1-1)
- 중복제거된 교집한 부분 사각형을 더해주면 된다. # sum(x1-1,y1-1)
- 답 : sum(x2,y2) - sum(x1-1,y2) - sum(x2,y1-1) + sum(x1-1,y1-1) #중복뺄셈보완


* 전체코드
#include <bits/stdc++.h>
using namespace std;
int n, m;
int arr[1025][1025], sum[1025][1025];
// sum[x][y] = (0,0)부터 (x,y)까지 사각형 안에 있는 수의 합
int sumAll;
int main() {
ios_base::sync_with_stdio(0);
cin.tie(0);
cin >> n >> m;
for (int i = 1; i <= n; ++i) {
for (int j = 1; j <= n; ++j) {
int tmp;
cin >> tmp;
arr[i][j] = tmp;
}
}
for (int i = 1; i <= n; ++i) {
for (int j = 1; j <= n; ++j) {
sum[i][j] = sum[i - 1][j] + sum[i][j - 1] - sum[i - 1][j - 1] + arr[i][j];
}
}
/*for (int i = 1; i <= n; ++i) {
for (int j = 1; j <= n; ++j) {
cout << sum[i][j] << " ";
}
cout << "\n";
}*/
int x1, x2, y1, y2;
for (int i = 0; i < m; ++i) {
cin >> x1 >> y1 >> x2 >> y2; // x2가 큰것은 보장됨.
cout << sum[x2][y2] - sum[x1 - 1][y2] - sum[x2][y1 - 1] + sum[x1 - 1][y1 - 1];
cout << "\n";
}
return 0;
}'Algorithm > 누적합' 카테고리의 다른 글
| [틀림] [알고리즘] 백준 2632 피자판매 // 누적합, 원형배열 (0) | 2025.02.22 |
|---|---|
| [알고리즘] 백준 10986 나머지합 // 누적합, 나누기 (0) | 2024.12.26 |